Probability of hypotheses. Bayes’s formulas.

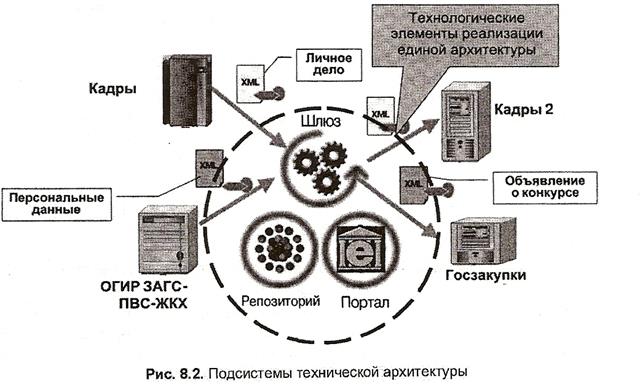
Let an event A can happen only in case of appearance of one of incompatible events B₁, B₂, …, B_n forming a complete group. Since it isn’t known beforehand which of these events will happen, we call them by hypotheses.

The probability of appearance of the event A is defined by the formula of total probability:

(*)

Assume that a trial has been made in result of which the event A was appeared. Pose the problem to determine how the probabilities of the hypotheses have been changed (in connection with that the event A has already happened). In other words, we will look for the conditional probabilities P_A(B₁), P_A(B₂), …, P_A(B_n). Find firstly the conditional probability P_A(B₁). By theorem of multiplication we have

Consequently,

Replacing P(A) by (*), we obtain

Formulas determining the conditional probabilities of the rest hypotheses are deduced analogously, i.e. the conditional probability of any hypothesis B_i (i = 1, 2, …, n) can be calculated by the formula

 

The obtained formulas are the Bayes’s formulas (on name of British mathematician who deduced them; published in 1764).

The Bayes’s formulas allow overestimating the probabilities of hypotheses after that a trial has been made in result of which the required event has appeared.

Example. Details produced by a factory shop are given for checking them on standard to one of two controllers. The probability that a detail will be given to the first controller is equal to 0,6; and to the second – 0,4. The probability that a suitable detail will be recognized standard by the first controller is equal to 0,94; and by the second controller – 0,98. A randomly chosen detail which is suitable has been recognized standard at checking. Find the probability that this detail was checked by the first controller.

Solution: Denote by A the event consisting in that a suitable detail has been recognized standard. One can make two assumptions: 1) The detail has been checked by the first controller (the hypothesis B ₁); 2) The detail has been checked by the second controller (the hypothesis B ₂).

Find the required probability that the detail has been checked by the first controller by the Bayes’s formula:

We have: P(B₁) = 0,6; P(B₂) = 0,4;

The required probability

We see the probability of the hypothesis B ₁ equals 0,6 before the trial, and after that a trial result has become known the probability of this hypothesis (more precise, the conditional probability) has been changed and become 0,59. Thus, using the Bayes’s formula allowed overestimating the probability of the considered hypothesis.

Glossary

compatible events – совместные события; hypothesis – гипотеза

beforehand – заранее; to recognize – признавать, распознавать

a factory shop – цех завода; suitable detail – годная деталь

to overestimate – переоценить

 

Exercises for Seminar 5

5.1. There are 20 skiers, 6 bicyclists and 4 runners in a group of sportsmen. The probability to execute the corresponding qualifying norm is: for a skier – 0,9, for a bicyclist – 0,8, and for a runner – 0,75. Find the probability that a randomly chosen sportsman will execute the norm.

The answer: 0,86.

 

5.2. The first box contains 20 details and 15 of them are standard; the second – 30 details and 24 of them are standard; the third – 10 details and 6 of them are standard. Find the probability that a randomly extracted detail from a randomly taken box is standard.

The answer: 43/60.

 

5.3. There are radio lamps in two boxes. The first box contains 12 lamps, and 1 of them is non-standard; the second box contains 10 lamps, and 1 of them is non-standard. A lamp is randomly taken from the first box and placed in the second. Find the probability that a randomly extracted lamp from the second box will be non-standard.

The answer: 13/132.

 

5.4. At a deviation of an automatic device from the normal operating mode the signaling device C-1 acts with the probability 0,8, and the signaling device C-11 acts with the probability 1. The probabilities that the automatic device is supplied with C-1 or C-11 are equal to 0,6 and 0,4 respectively. A signal about cutting the automatic device has been received. What is more probable: the automatic device is supplied with the signaling device C-1 or C-11?

The answer: The probability that the automatic device is supplied with C-1, is equal to 6/11, and C-11 – 5/11.

 

5.5. The probability for products of a certain factory to satisfy the standard is equal to 0,96. A simplified system of checking on standardness gives positive result with the probability 0,98 for products satisfying the standard, and with the probability 0,05 – for products non-satisfying the standard. A randomly taken product has been recognized as standard at checking. Find the probability that it really satisfies the standard.

The answer: 0,998.

 

5.6. A digit is firstly randomly chosen from the digits {1, 2, 3, 4, 5}, and then the second digit – from the rest four digits. Find the probability that an odd digit will be chosen:

a) for the first time; b) for the second time; c) in both times.

The answer: a) 3/5; b) 3/5; c) 3/10.

 

5.7. Three cards are randomly selected without replacement from an ordinary deck of 52 playing cards. Compute the conditional probability that the first card selected is a spade, given that the second and third cards are spades.

The answer: 0,22.

 

5.8. Urn A contains 2 white balls and 1 black ball, whereas urn B contains 1white ball and 5 black balls. A ball is drawn at random from urn A and placed in urn B. A ball is then drawn from urn B. It happens to be white. What is the probability that the ball transferred was white?

The answer: 0,8.

 

Exercises for Homework 5

5.9. A collector has received 3 boxes of details made by the factory № 1, and 2 boxes of details made by the factory № 2. The probability that a detail of the factory № 1 is standard is equal to 0,8, and the factory № 2 – 0,9. The collector has randomly extracted a detail from a randomly taken box. Find the probability that a standard detail has been extracted (a collector – сборщик).

The answer: 0,84.

 

5.10. There are 4 kinescopes in a television studio. The probabilities that the kinescope will sustain the warranty period of service are equal to 0,8; 0,85; 0,9; 0,95 respectively. Find the probability that a randomly taken kinescope will sustain the warranty period of service (to sustain – выдержать).

The answer: 0,875.

 

5.11. A die has been randomly extracted from the full set of 28 dice of domino. Find the probability that the second randomly extracted die can be put to the first.

The answer: 7/18.

 

5.12. For participation in student selective sport competitions 4 students has been directed from the first group, 6 – from the second, 5 – from the third group. The probabilities that a student of the first, second and third group gets in the combined team of institute, are equal to 0,9; 0,7 and 0,8 respectively. A randomly chosen student as a result of competition has got in the combined team. Which of groups is this student most likely belonged to (a combined team – сборная)?

The answer: The probabilities that the student has been chosen from the first, second and third group are equal to 18/59, 21/59, 20/59 respectively.

5.13. English and American spellings are rigour and rigor, respectively. A man staying at a Parisian hotel writes this word, and a letter taken at random from his spelling is found to be a vowel. If 40 percent of the English-speaking men at the hotel are English and 60 percent are Americans, what is the probability that the writer is an Englishman (rigour (rigor) – суровость; a vowel – гласная)?

The answer: 5/11.

 

5.14. Urn A has 5 white and 7 black balls. Urn B has 3 white and 12 black balls. We flip a coin. If the outcome is heads, then a ball from urn A is selected, whereas if the outcome is tails, then a ball from urn B is selected. Suppose that a white ball is selected. What is the probability that the coin landed on tails (to flip – подбросить)?

The answer: 12/37.

 

5.15. There are four urns. The first urn contains 1 white and 1 black ball, the second – 2 white and 3 black balls, the third – 3 white and 5 black balls, and the fourth – 4 white and 7 black balls. The event H_i is the choosing the i -th urn (i = 1, 2, 3, 4). It is known that the probability of choosing the i -th urn is equal to i /10. A ball is randomly extracted from a randomly chosen urn. Find the probability that a randomly extracted ball is white.

The answer: 0,388.

 

L E C T U R E 6